Page 1 - HA-Tangents Ch-10 (Circles)

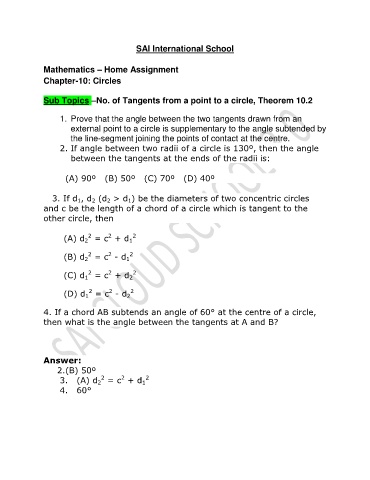
SAI International School

Mathematics – Home Assignment

Chapter-10: Circles

Sub Topics –No. of Tangents from a point to a circle, Theorem 10.2

1. Prove that the angle between the two tangents drawn from an
external point to a circle is supplementary to the angle subtended by
the line-segment joining the points of contact at the centre.
2. If angle between two radii of a circle is 130º, then the angle
between the tangents at the ends of the radii is:

(A) 90º (B) 50º (C) 70º (D) 40º

3. If d , d (d > d ) be the diameters of two concentric circles
2
1
2
1
and c be the length of a chord of a circle which is tangent to the
other circle, then
2
2
2
(A) d = c + d
2
1
2
2
2
(B) d = c - d
2
1
2
2
2
(C) d = c + d
2
1
2
2
2
(D) d = c - d
1
2
4. If a chord AB subtends an angle of 60° at the centre of a circle,
then what is the angle between the tangents at A and B?

Answer:
2.(B) 50º
2
2
3. (A) d = c + d 1 2
2
4. 60°